14 Formal vs. Empirical Sciences

The distinction between analytic and synthetic propositions comes in handy when distinguishing between formal and empirical sciences. While in empirical sciences, such as physics, chemistry, biology, psychology, sociology, or economics, there are both analytic and synthetic propositions, in formal sciences, such as logic or mathematics, all propositions are analytic. It is the absence of synthetic propositions that characterizes formal sciences; indeed, all propositions of math or logic are either definitions or follow from definitions.

Even those mathematical propositions that don’t really strike us as analytic are essentially analytic. Consider, for instance, Euclid’s famous postulate:

• The sum of the angles of a triangle is two right angles.

It is not quite obvious that this postulate is analytic, since it is possible to negate it. In fact, Euclid’s postulate has no place in the non-Euclidean hyperbolic geometry of Lobachevsky, where the sum of the angles of a triangle is less than 180°, as well as in the non-Euclidean elliptical geometry of Riemann, where the sum of the angles of a triangle is greater than 180°:

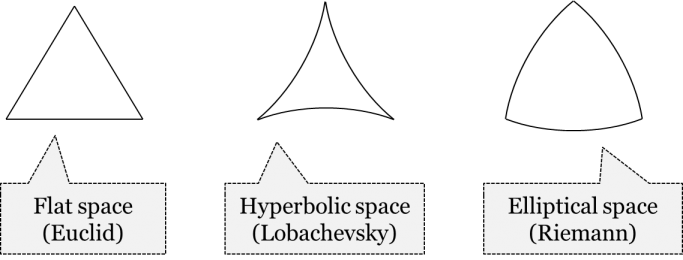
But if it is possible to negate some mathematical propositions, then in what sense are they said to be analytic propositions? The answer is this: while some mathematical propositions don’t usually strike us as definitions, they essentially define properties of mathematical objects that are studied by that specific mathematical theory, and thus are veiled definitions. What makes this possible is the fact that the objects of mathematical theories (such as triangles) are all formal, i.e. they do not exist independently of those mathematical theories that define them with their postulates and definitions. Thus, Euclid’s postulate remains an analytic proposition in the context of Euclidean geometry, whereas some other postulates would be analytic in Lobachevsky’s and Riemann’s non-Euclidean geometries. The same goes for the objects of any formal science.

In contrast, theories of empirical science don’t have the luxury of defining the properties of their objects. The objects of empirical science – natural or social – are all generally assumed to exist independently of the empirical theories that attempt to describe them. Thus, physical processes described by a physical theory are not defined/created by the physical theory. Similarly, a biological theory attempts to describe plants and animals that exist independently of that theory – the biological theory does not define/create organisms. By the same token, theories in political science try to describe political processes, like the succession of a new monarch, which would have occurred even if there were no political science.

Because the objects of empirical theories are not defined by empirical theories themselves, empirical theories cannot consist only of analytic propositions. They must contain at least some synthetic propositions that are hypotheses about the objects under study. For example, a physical theory cannot merely give us the definitions of “force of gravity”, “mass”, or “distance”, without telling us how mass and distance affect the force of gravity. Similarly, a biological theory doesn’t merely tell us what it means by “species” or “evolution’, but, importantly, tells us how species evolve through time. It is these relations between different features of natural or social objects (structures, systems, processes, etc.) that any empirical theory attempts to uncover. Thus, hypotheses about these objects, i.e. synthetic propositions, are inevitable in empirical science.

The rule of thumb for distinguishing formal from empirical theories is this: if a theory contains no synthetic propositions, it is a formal theory; if a theory contains at least one synthetic proposition, it is an empirical theory. If we consider any theory from any one of the plethora of empirical sciences, we will see that it contains some synthetic propositions, i.e. propositions the opposite of which is conceivable. We’ve already seen this in the example of the law of gravity. The same applies to the chemical formula for the combustion of methane – it is possible to conceive of a world with a different set of chemical laws that make methane combust differently, or even not combust at all. The same applies to all synthetic propositions of all empirical sciences.